Next: Problem with earlier simple Up: Quarks and other Elementary Previous: Further observations on phase Contents
Recall above as shown in Figure 2 that a "trick" had to be deployed - the "decay" equation altered so that two particles (neutron, neutrino) were phase-shifted into two others (proton, electron). The reason for showing this as a first example was because attempts to do the "traditional" neutron-proton decay proved extraordinarily convoluted, and have yet to be solved.
Which leads to a thought: if neutron "decay" (from one particle to three) is so complex as to be extremely challenging even to solve, but the phase-shift of neutron-neutrino (two particles) into proton-electron (two particles) is so simple, then what really is going on? Using Occam's Razor we conclude that "decay" of one particle into three - even via a W- Boson - is not what's actually happening, inference from the Standard Model to the contrary. Perhaps however that complexity is actually genuinely there, which would go part-way to explaining why a neutron has such a long half-life.
After careful and considerable endeavours to resolve both sides of the equation in terms of Rishon phase-shift rules, the following diagram was the closest approximation which could be achieved:
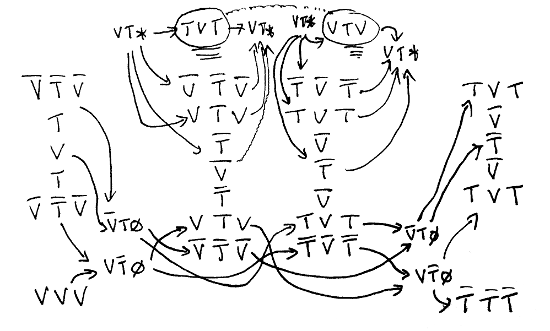figureNeutron genuinely does not fit the rules.
Culprit: decay energy
|
On the left side is the neutron and underneath a neutrino. On the right is the proton and underneath it the electron. In the middle is a W- Boson: above is the temporary introduction of a Pion+. Bear in mind that the W- Boson is considered to be the "Weak Force" i.e. the two ultra-quarks involved have, unlike the "gluon", a definite lifetime. Note when comparing this diagram against the "simple" version, it is the four quarks at the bottom-middle that are utilised to indicate temporary carriers of the neutron and neutrino as it phase-transitions into a proton and electron.
The mess at the top indicates two sets of VT* transforms. Each creating-VT* represent the creation of each of the ultra-quarks. When the W- Boson decays, two more VT* events occur which result in their erasure. The problem is: whilst the four quarks at the bottom are accounted for, the six remaining quarks cannot be neatly accounted for. The discrepancy can only be balanced, in Rishon phase-change rules, through the addition of a second particle: a Pion+.
Whilst there may be other ways in which this can be expressed, in effect the violation of the rules has a cost (an energy cost). In fact, there is exactly that: 0.782MeV of energy cost, known as the "decay energy".
The above numerical coincidence gives a finger-in-the-air figure indicating that there is some relationship here that has previously not been noticed. What is noteworthy however is that whilst the above decay diagram may not necessarily be a completely accurate representation of the underlying process, the fact that there is no clean Rishon phase transition possible, and the fact that the neutron's decay results in 0.782MeV unaccountable energy is not a coincidence.
In conclusion, then: we have the first transformation which can actually be said to genuinely be a "decay".